Introduction
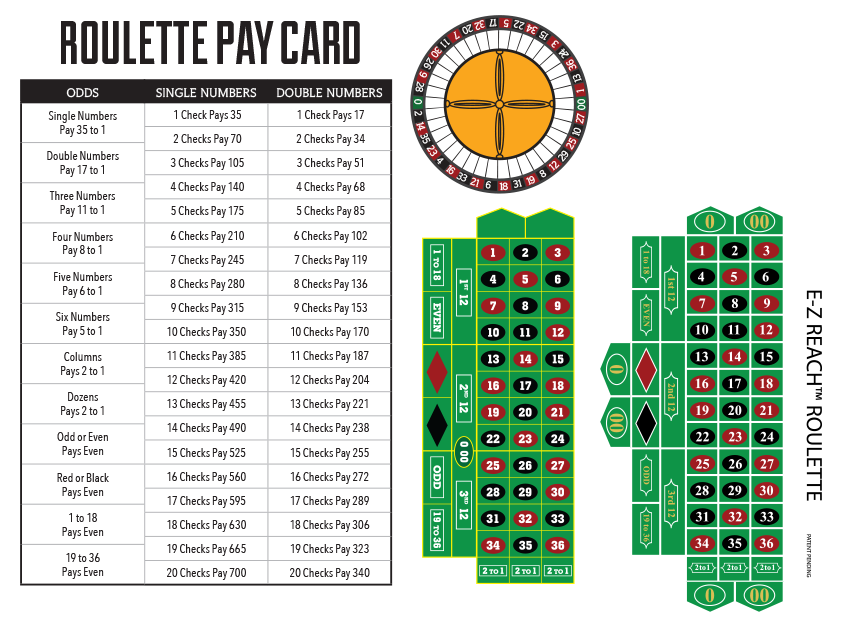
Odd or Even – This is a bet on whether the ball will land on an even number or an odd one. The chances of winning this bet are 48.65% (18 in 37) and 47.37% (18 in 38) for European and American Roulette, respectively, while the payout is 1:1. Roulette is a drain on your wallet simply because the game doesn't pay what the bets are worth. With 38 numbers (1 to 36, plus 0 and 00), the true odds of hitting a single number on a straight-up bet are 37 to 1, but the house pays only 35 to 1 if you win! Ditto the payouts on the combination bets.
The Gambler's Fallacy is the mistaken belief that if an independent event has not happened in a long time, then it becomes overdue and more likely. It is also equally incorrect that if an outcome has happened a disproportionate number of times lately, compared to statistical expectations, then it becomes overheated and less likely to occur the next time. An example of this fallacious thinking might be that if the number 23 hasn't been drawn in a 6-49 lottery the last 100 games, then it becomes more likely to be drawn during the next drawing.
Many worthless betting strategies and systems are based on belief in the Gambler's Fallacy. I got the idea for writing about this after reading an 888 online roulette article by Frank Scoblete entitled How to Take Advantage of Roulette Hot Spots. In that article, Scoblete recommends taking a count of each outcome for 3,700 spins in single-zero roulette and 3,800 spins in double-zero roulette in the hunt for 'hot numbers.' Never mind that this would take about 100 hours to make this many observations, assuming the industry standard of 38 spins per hour.
Before going further, let me say that I strongly believe modern roulette wheels made by top brands like Cammegh are extremely precise and any bias would be minuscule compared to the house advantage. Thus, testing a modern roulette for bias would be a total waste of time. Now, testing a 30-year-old hand-me-down wheel in a banana republic might be another story. However, you're on your own if you win a lot of money from said casino and try to leave with it.
That said, if you track 3,800 outcomes in single-zero roulette, the average number of times any number will hit is 3800/38=100. I ran a simulation of over 1.3 trillion spins, counting how many times each number was hit, sorting the outcomes to find the most frequent number and how many times it was observed, and keeping a count of how many times the most frequent number in each simulation was seen.
Hottest Number in 3,800 Spins of Double-Zero Roulette
As a former actuary, I hate to use a layman's term like the 'hottest number,' but that is how gamblers talk so will go with that. That said, following are the results of the count of the hottest number in millions of 3800-spin simulations.
Count of the Hottest Number in 3,800 Spins on Double-Zero Wheel
| Statistic | Value |
|---|---|
| Mean | 122.02 |
| Median | 121 |
| Mode | 120 |
| 90th Percentile | 128 |
| 95th Percentile | 131 |
| 99th Percentile | 136 |
| 99.9th Percentile | 142 |
Here is what the table above means in plain simple English.
- The mean, or average, count of the hottest number is 122.02.
- The median count of the most frequent number is 121. This means that over 50% of time the most frequent number appeared 121 times or less, as well as 121 times or more. This is possible because the probability of 121 observations is in both groups.
- The mode, or most count of the hottest number is 120, which happens 8.29% of the time.
- The 90th percentile is the smallest number such that the probability the count of the hottest number is at least 90% .
- The 95th percentile is the smallest number such that the probability the count of the hottest number is at least 95%.
- The 99th percentile is the smallest number such that the probability the count of the hottest number is at least 99%.
- The 99.9th percentile is the smallest number such that the probability the count of the hottest number is at least 99.9%.
Hottest Number in 3,700 Spins of Single-Zero Roulette
The results are very similar with 3,700 spins tracked on a single-zero wheel. Following is a summary of the results.
Count of the Hottest Number in 3,700 Spins on Single-Zero Wheel
| Statistic | Value |
|---|---|
| Mean | 121.90 |
| Median | 121 |
| Mode | 120 |
| 90th Percentile | 128 |
| 95th Percentile | 131 |
| 99th Percentile | 136 |
| 99.9th Percentile | 142 |
The following table shows the full results of the simulation on both wheels. The two commulative columns show the probability that the count of the hottest number is the number on the left column or more. For example, the probability the hottest number in 3,700 spins of single-zero roulette is 130 or more is 0.072044.
Summary of the Count of the Hottest Number in 3,700 Spins of Single-Zero Roulette and 3,800 spins of Double-Zero Roulette
| Count | Probability Single Zero | Cummulative Single Zero | Probability Double Zero | Cummulative Double Zero |
|---|---|---|---|---|
| 160 or More | 0.000001 | 0.000001 | 0.000001 | 0.000001 |
| 159 | 0.000000 | 0.000001 | 0.000000 | 0.000001 |
| 158 | 0.000001 | 0.000001 | 0.000001 | 0.000001 |
| 157 | 0.000001 | 0.000002 | 0.000001 | 0.000002 |
| 156 | 0.000001 | 0.000003 | 0.000001 | 0.000003 |
| 155 | 0.000002 | 0.000005 | 0.000002 | 0.000005 |
| 154 | 0.000003 | 0.000009 | 0.000003 | 0.000008 |
| 153 | 0.000005 | 0.000013 | 0.000005 | 0.000013 |
| 152 | 0.000007 | 0.000020 | 0.000008 | 0.000021 |
| 151 | 0.000012 | 0.000032 | 0.000012 | 0.000033 |
| 150 | 0.000017 | 0.000049 | 0.000018 | 0.000051 |
| 149 | 0.000026 | 0.000075 | 0.000027 | 0.000077 |
| 148 | 0.000038 | 0.000114 | 0.000041 | 0.000118 |
| 147 | 0.000060 | 0.000174 | 0.000062 | 0.000180 |
| 146 | 0.000091 | 0.000265 | 0.000092 | 0.000273 |
| 145 | 0.000132 | 0.000397 | 0.000137 | 0.000409 |
| 144 | 0.000195 | 0.000592 | 0.000199 | 0.000608 |
| 143 | 0.000282 | 0.000874 | 0.000289 | 0.000898 |
| 142 | 0.000409 | 0.001283 | 0.000421 | 0.001319 |
| 141 | 0.000580 | 0.001863 | 0.000606 | 0.001925 |
| 140 | 0.000833 | 0.002696 | 0.000860 | 0.002784 |
| 139 | 0.001186 | 0.003882 | 0.001215 | 0.003999 |
| 138 | 0.001652 | 0.005534 | 0.001704 | 0.005703 |
| 137 | 0.002315 | 0.007849 | 0.002374 | 0.008077 |
| 136 | 0.003175 | 0.011023 | 0.003286 | 0.011363 |
| 135 | 0.004355 | 0.015378 | 0.004489 | 0.015852 |
| 134 | 0.005916 | 0.021295 | 0.006088 | 0.021940 |
| 133 | 0.007939 | 0.029233 | 0.008196 | 0.030136 |
| 132 | 0.010601 | 0.039834 | 0.010908 | 0.041044 |
| 131 | 0.013991 | 0.053824 | 0.014384 | 0.055428 |
| 130 | 0.018220 | 0.072044 | 0.018757 | 0.074185 |
| 129 | 0.023498 | 0.095542 | 0.024114 | 0.098299 |
| 128 | 0.029866 | 0.125408 | 0.030603 | 0.128901 |
| 127 | 0.037288 | 0.162696 | 0.038228 | 0.167130 |
| 126 | 0.045771 | 0.208467 | 0.046898 | 0.214027 |
| 125 | 0.055165 | 0.263632 | 0.056310 | 0.270337 |
| 124 | 0.064853 | 0.328485 | 0.066020 | 0.336357 |
| 123 | 0.074178 | 0.402662 | 0.075236 | 0.411593 |
| 122 | 0.081929 | 0.484591 | 0.082885 | 0.494479 |
| 121 | 0.087158 | 0.571750 | 0.087696 | 0.582174 |
| 120 | 0.088520 | 0.660269 | 0.088559 | 0.670734 |
| 119 | 0.084982 | 0.745252 | 0.084406 | 0.755140 |
| 118 | 0.076454 | 0.821705 | 0.075245 | 0.830385 |
| 117 | 0.063606 | 0.885312 | 0.061851 | 0.892236 |
| 116 | 0.048069 | 0.933381 | 0.046111 | 0.938347 |
| 115 | 0.032432 | 0.965813 | 0.030604 | 0.968952 |
| 114 | 0.019117 | 0.984930 | 0.017664 | 0.986616 |
| 113 | 0.009567 | 0.994496 | 0.008614 | 0.995230 |
| 112 | 0.003894 | 0.998390 | 0.003420 | 0.998650 |
| 111 | 0.001257 | 0.999647 | 0.001065 | 0.999715 |
| 110 | 0.000297 | 0.999944 | 0.000243 | 0.999958 |
| 109 | 0.000050 | 0.999994 | 0.000038 | 0.999996 |
| 108 or Less | 0.000006 | 1.000000 | 0.000004 | 1.000000 |
Count of the Hottest Numbers in 300 Spins in Double-Zero Roulette
What if you don't want to spend 100 hours gathering data on a single wheel? Some casinos are kind enough to give you, on a silver platter, the number of times in the last 300 spins the four 'hottest' and 'coolest' numbers occurred. The image at the top of the page shows an example taken on a double-zero wheel at the Venetian.
In 300 spins, the average number of wins on a double-zero wheel for any number is 300/38=7.9. As you can see from the image above, the four hottest numbers were 20, 5, 29, and 2, which occurred 15, 14, 13, and 12 times respectively. Is this unusual? No. In a simulation of over 80 billion spins, the most frequent number, in 300-spin experiments, appeared most frequently at 14 times with a probability of 27.4%. The most likely total of the second, third, and fourth most frequent numbers was 13, 12, and 12 times respectively, with probabilities of 37.9%, 46.5%, and 45.8%. So the results of the 'hottest' numbers in the image above were a little more flat than average.
The following table shows the probabilities of the four hottest numbers in 300 spins of double-zero roulette. For example, the probability the third most frequent number happens 15 times is 0.009210.
Count of the Hottest Four Numbers in 300 Spins on a Double-Zero Wheel
| Observations | Probability Most Frequent | Probability Second Most Frequent | Probability Third Most Frequent | Probability Fourth Most Frequent |
|---|---|---|---|---|
| 25 or More | 0.000022 | 0.000000 | 0.000000 | 0.000000 |
| 24 | 0.000051 | 0.000000 | 0.000000 | 0.000000 |
| 23 | 0.000166 | 0.000000 | 0.000000 | 0.000000 |
| 22 | 0.000509 | 0.000000 | 0.000000 | 0.000000 |
| 21 | 0.001494 | 0.000001 | 0.000000 | 0.000000 |
| 20 | 0.004120 | 0.000009 | 0.000000 | 0.000000 |
| 19 | 0.010806 | 0.000075 | 0.000000 | 0.000000 |
| 18 | 0.026599 | 0.000532 | 0.000003 | 0.000000 |
| 17 | 0.060526 | 0.003263 | 0.000060 | 0.000001 |
| 16 | 0.123564 | 0.016988 | 0.000852 | 0.000020 |
| 15 | 0.212699 | 0.071262 | 0.009210 | 0.000598 |
| 14 | 0.274118 | 0.215025 | 0.068242 | 0.011476 |
| 13 | 0.212781 | 0.379097 | 0.283768 | 0.117786 |
| 12 | 0.067913 | 0.270747 | 0.464748 | 0.457655 |
| 11 | 0.004615 | 0.042552 | 0.168285 | 0.383900 |
| 10 | 0.000017 | 0.000448 | 0.004830 | 0.028544 |
| 9 | 0.000000 | 0.000000 | 0.000001 | 0.000020 |
| Total | 1.000000 | 1.000000 | 1.000000 | 1.000000 |
The next table shows the mean, median, and mode for the count of the first, second, third, and fourth hottest numbers in millions of 300-spin simulations of double-zero roulette.
Summary of the Count of the Four Most Frequent Numbers in 300 Spins of Double-Zero Wheel
| Order | Mean | Median | Mode |
|---|---|---|---|
| First | 14.48 | 14 | 14 |
| Second | 13.07 | 13 | 13 |
| Third | 12.27 | 12 | 12 |
| Fourth | 11.70 | 12 | 12 |
Count of the Coolest Numbers in 300 Spins in Double-Zero Roulette
The next table shows the probability of each count of the four collest numbers in 300 spins of double-zero roulette.
Count of the Coolest Four Numbers in 300 Spins on a Double-Zero Wheel
| Observations | Probability Least Frequent | Probability Second Least Frequent | Probability Third Least Frequent | Probability Fourth Least Frequent |
|---|---|---|---|---|
| 0 | 0.012679 | 0.000063 | 0.000000 | 0.000000 |
| 1 | 0.098030 | 0.005175 | 0.000135 | 0.000002 |
| 2 | 0.315884 | 0.088509 | 0.012041 | 0.001006 |
| 3 | 0.416254 | 0.420491 | 0.205303 | 0.063065 |
| 4 | 0.150220 | 0.432638 | 0.595139 | 0.522489 |
| 5 | 0.006924 | 0.052945 | 0.185505 | 0.401903 |
| 6 | 0.000008 | 0.000180 | 0.001878 | 0.011534 |
| Total | 1.000000 | 1.000000 | 1.000000 | 1.000000 |
The next table shows the mean, median, and mode for the count of the first, second, third, and fourth coolest numbers in the 300-spin simulations of double-zero roulette.
Summary of the count of the Four Least Frequent Numbers on a Double-Zero Wheel
| Order | Mean | Median | Mode |
|---|---|---|---|
| Least | 2.61 | 3 | 3 |
| Second Least | 3.44 | 3 | 4 |
| Third Least | 3.96 | 4 | 4 |
| Fourth Least | 4.36 | 4 | 4 |
Count of the Hottest Numbers in 300 Spins of Single-Zero Roulette
In 300 spins, the average number of wins on a single-zero wheel for any number is 300/37=8.11. The next table shows the probability of each count of the four coolest numbers in 300 spins of double-zero roulette. For example, the probability the third most frequent number happens 15 times is 0.015727.
Count of the Hottest Four Numbers in 300 Spins on a Single-Zero Wheel
| Observations | Probability Most Frequent | Probability Second Most Frequent | Probability Third Most Frequent | Probability Fourth Most Frequent |
|---|---|---|---|---|
| 25 or More | 0.000034 | 0.000000 | 0.000000 | 0.000000 |
| 24 | 0.000078 | 0.000000 | 0.000000 | 0.000000 |
| 23 | 0.000245 | 0.000000 | 0.000000 | 0.000000 |
| 22 | 0.000728 | 0.000000 | 0.000000 | 0.000000 |
| 21 | 0.002069 | 0.000002 | 0.000000 | 0.000000 |
| 20 | 0.005570 | 0.000018 | 0.000000 | 0.000000 |
| 19 | 0.014191 | 0.000135 | 0.000000 | 0.000000 |
| 18 | 0.033833 | 0.000905 | 0.000008 | 0.000000 |
| 17 | 0.074235 | 0.005202 | 0.000125 | 0.000001 |
| 16 | 0.144490 | 0.025286 | 0.001624 | 0.000050 |
| 15 | 0.232429 | 0.097046 | 0.015727 | 0.001286 |
| 14 | 0.269735 | 0.259360 | 0.101259 | 0.021054 |
| 13 | 0.177216 | 0.382432 | 0.347102 | 0.175177 |
| 12 | 0.043266 | 0.208137 | 0.429715 | 0.508292 |
| 11 | 0.001879 | 0.021373 | 0.102979 | 0.283088 |
| 10 | 0.000003 | 0.000103 | 0.001461 | 0.011049 |
| 9 | 0.000000 | 0.000000 | 0.000000 | 0.000002 |
| Total | 1.000000 | 1.000000 | 1.000000 | 1.000000 |
The next table shows the mean, median, and mode for the count of the first, second, third, and fourth hottest numbers in millions of 300-spin simulations of double-zero roulette.
Summary — Count of the Four Hottest Numbers — Double-Zero Wheel
| Order | Mean | Median | Mode |
|---|---|---|---|
| First | 14.74 | 15 | 14 |
| Second | 13.30 | 13 | 13 |
| Third | 12.50 | 12 | 12 |
| Fourth | 11.92 | 12 | 12 |
Count of the Coolest Numbers in 300 Spins in Single-Zero Roulette
The next table shows the probability of each count of the four coolest numbers in 300 spins of double-zero roulette. For example, the probability the third coolest numbers will be observed five times is 0.287435.
Count of the Coolest Four Numbers in 300 Spins on a Double-Zero Wheel
| Observations | Probability Least Frequent | Probability Second Least Frequent | Probability Third Least Frequent | Probability Fourth Least Frequent |
|---|---|---|---|---|
| 0 | 0.009926 | 0.000038 | 0.000000 | 0.000000 |
| 1 | 0.079654 | 0.003324 | 0.000068 | 0.000001 |
| 2 | 0.275226 | 0.062392 | 0.006791 | 0.000448 |
| 3 | 0.419384 | 0.350408 | 0.140173 | 0.034850 |
| 4 | 0.200196 | 0.484357 | 0.557907 | 0.406702 |
| 5 | 0.015563 | 0.098547 | 0.287435 | 0.521238 |
| 6 | 0.000050 | 0.000933 | 0.007626 | 0.036748 |
| 7 | 0.000000 | 0.000000 | 0.000001 | 0.000013 |
| Total | 1.000000 | 1.000000 | 1.000000 | 1.000000 |
The next table shows the mean, median, and mode for the count of the first, second, third, and fourth coolest numbers in the 300-spin simulations of single-zero roulette.
Summary of the count of the Four Least Frequent Numbers on a Single-Zero Wheel
| Order | Mean | Median | Mode |
|---|---|---|---|
| Least | 2.77 | 3 | 3 |
| Second Least | 3.62 | 4 | 4 |
| Third Least | 4.15 | 4 | 4 |
| Fourth Least | 4.56 | 5 | 5 |
The least I hope you have learned from this article is it is to be expected that certain numbers will come up more than others. To put it in other words, it is natural that some numbers will be 'hot' and some 'cool.' In fact, such differences from the mean are highly predictable. Unfortunately, for roulette players, we don't know which numbers will be 'hot,' just that some of them almost certainly will be. I would also like to emphasize, contrary to the Gambler's Fallacy, that on a fair roulette wheel that every number is equally likely every spin and it makes no difference what has happened in the past.
Finally, it should not be interpreted that we give an endorsement to the 888 Casino, which we linked to earlier. I am very bothered by this rule in their rule 6.2.B. Before getting to that, let me preface with a quote from rule 6.1, which I'm fine with.
'If we reasonably determine that you are engaging in or have engaged in fraudulent or unlawful activity or conducted any prohibited transaction (including money laundering) under the laws of any jurisdiction that applies to you (examples of which are set out at section 6.2 below), any such act will be considered as a material breach of this User Agreement by you. In such case we may close your account and terminate the User Agreement in accordance with section 14 below and we are under no obligation to refund to you any deposits, winnings or funds in your account.' -- Rule 6.1
Let's go further now:
The following are some examples of 'fraudulent or unlawful activity' -- Rule 6.2
Next, here is one of many examples listed as rule 6.2.B
'Unfair Betting Techniques: Utilising any recognised betting techniques to circumvent the standard house edge in our games, which includes but is not limited to martingale betting strategies, card counting as well as low risk betting in roulette such as betting on red/black in equal amounts.' -- Rule 6.2.B
Let me make it perfectly clear that all betting systems, including the Martingale, not only can't circumvent the house edge, they can't even dent it. It is very mathematically ignorant on the part of the casino to fear any betting system. Why would any player trust this casino when the casino can seize all their money under the reason that the player was using a betting system? Any form of betting could be called a betting system, including flat betting. Casino 888 normally has a pretty good reputation, so I'm surprised they would lower themselves to this kind of rogue rule.
Written by: Michael Shackleford
By Ion Saliu, Founder of Roulette Mathematics
This question was posted in mathematical newsgroups (alt.math.recreational, alt.math.undergrad, alt.sci.math.probability): 'Winning and Quitting on Red/Black in Roulette'.
- 'Obviously in roulette betting on in the long run you are going to lose your money but at some point chances are you'll be in profit. To take an extreme example if you had $1000 you could reasonably expect to be up $1 at some point. Is it possible to generalize this? I want to win W dollars at which point I will quit. How much cash C would I need to have probability P of succeeding? Let's say I'm betting on a 37 number roulette wheel (18 red 18 black and one green 0)?'
On the surface, the best probability for the roulette player to be ahead is in one trial (spin): 48.6% to win (versus 51.4% to lose), as far as even-money betting is concerned. I don't agree that it is the best strategy (betting all your bankroll on one spin).
Theoretically, no bankroll will put a player ahead guaranteed, IF flat-betting and playing very long consecutive sessions. There are moments, however, when the roulette player can be ahead by at least one betting unit. Even in even-money bets, the player has a good chance to be ahead by at least one unit after 5, or 10, or even 100 spins. But more than 20 spins are NOT recommended; the probability (odds) to lose go(es) above 50%! Think about it!
The main thing, mathematically, is the number of player's wins in N trials. To be ahead, means the player has won at least one more roulette spin (number of successes) than the number of losses in N trials. The question then becomes:
'What are the probabilities for the player to be ahead in various numbers of trials?'
Everybody can use my probability software SuperFormula, option L: At Least M successes in N trials.
Winning probability: p = 18/37; M must be at least (N/2) + 1.
Here is a number of cases from the player's perspective.
The figures are applicable to all even-money roulette bets: black or red; even or odd; low or high (1-18 or 19-36).
1 trial (spin)
- probability (odds) to win: 48.6%; odds = 1 in 2.05
- probability (odds) to lose: 51.4%; odds = 1 in 1.95
(the probability to lose is 19/37; adding zero to unfavorable cases).
2 trials (spins)
- probability (odds) to win 2 of 2: 23.7% (1 of 2 doesn't mean 'being ahead')
- probability (odds) to lose 1 of 2: 76.3%
3 spins
- probability (odds) to win at least 2 of 3: 48%
- probability (odds) to lose at least 2 of 3: 52%
10 spins
- probability (odds) to win at least 6 of 10: 34.4%; odds = 1 in 2.91
- probability (odds) to lose at least 6 of 10: 41.1%; odds = 1 in 2.43
20 spins
- probability (odds) to win at least 11 of 20: 36.5%
- probability (odds) to lose at least 11 of 20: 46.2%
Roulette Odds Calculator
100 spins
- probability (odds) to win at least 51 of 100: 35.5%; odds = 1 in 2.82
- probability (odds) to lose at least 51 of 100: 56.8%; odds = 1 in 1.76.
It's getting worse for the player...
The roulette strategy (or system) is a totally different ball game! But there are professional gamblers out there, including roulette players! They must have strategies, some roulette systems deduced from some figures like the ones above! The player can be ahead at any point in the game. If so, maybe it's time to move to another (or casino) table: It improves the odds of winning!
Always keep track of the losing and winning streaks. Be strong and put an end to a winning streak. You are ahead, you quit the roulette table. Go to another table and wait until you are ahead. The bankroll is of the essence: It must assure going through long losing streaks. Divide the streaks in 10 spins or 20 spins. Never fight aggressively short or mid-term losing streaks. This is the best approach for those who do not know Ion Saliu's casino gambling systems. A good approach to gambling is the next best thing to a good gambling system! Applicable to blackjack and baccarat, too!
Axiomatic one, everybody knows that the casinos have an edge or house advantage (HA) in all the games they offer, roulette including. The house advantage is created by the payouts in rapport to total possibilities for the respective bet. We can apply this simple formula based on units paid UP over total possibilities TP:
HA = 1 – (UP / TP)(always expressed as a percentage.)
For example, in single-zero roulette, the one-number (straight-up) bet has payout of 35 to 1. The to qualifier is very important: the casino pays you 35 units and they give you back the unit you bet; thus, you get 36 units. There are 37 possibilities in single-zero roulette: 36 numbers from 1 to 36 plus the 0 number. Therefore, HA = 1 – (UP / TP) = 1 – (36 / 37) = 1 – 0.973 = 0.027 = 2.7%.
Let's calculate HA for the 1 to 1 bets: black/red, even/odd, low/high. HA = 1 – (UP / TP) = 1 – (2 / 2.055) = 1 – 0.973 = 0.027 = 2.7%. There are little differences among bets depending on how many decimal points we work with in our calculations.
The point is, the casinos have an advantage, or the players have a disadvantage. Nonetheless, the players' disadvantage is far better than what they face in state-run lotteries. Yet, most casino gamblers lose big, including at roulette tables. They do not have sufficient bankrolls to withstand long losing streaks.
However, around 45% of the roulette numbers lead the gamblers to profits in a few thousand spins. That is, with a sufficient bankroll, a player has a pretty good chance to make a profit, even if playing a random roulette number, or a favorite number. I analyzed about 8000 roulette spins from Hamburg Spielbank (casino). Quite a few numbers ended up making a profit: roulette systems, magic numbers.
By contrast, the more lottery drawings a player plays, the higher the degree of certainty of a loss. Let's make a comparative analysis to the roulette long series above (spins: total roulette numbers, 37, multiplied by 200). If playing the pick-3 lottery for some 100,000 drawings, it is guaranteed that all pick-3 straight sets will be losers. Some numbers will hit up to 3% to 5% above the norm — but that is not nearly enough to assure a profit. A frequency of 3% to 5% above the norm leads to profits in roulette, however.
Ion Saliu's Paradox and Roulette
Ion Saliu's Paradox of N TrialsRoulette Number Odds By Mike Lindell
is presented in detail at saliu.com, especially the probability theory page and the mathematics of gambling formula. If p = 1 / NNumber Layout Roulette Wheel
, we can discover an interesting relation between the degree of certainty DC and the number of trials N. The degree of certainty has a limit, when N tends to infinity. That limit is 1 — 1/e, or approximately 0.632....If you play 1 roulette number for the next 38 spins, common belief was that you expected to win once. Not! Non! Only if you play 38 numbers in 1 spin, your chance to hit the winning number is 100%. Here is an interesting table, which includes also The Free Roulette System #1 presented at the main roulette site.
The maximum gain comes when playing 38 numbers in one spin: 36.3%. Obviously, it makes no sense to play that way because of the house advantage. On the other hand, a so-called wise gambler is more than happy to play one number at a time. What he does is simply losing slowly! Not only that, but losing slowly is accompanied by losing more. That cautious type of gambling is like a placebo. A roulette system such as Free System #1 scares most gamblers. 'Play 34 or 33 numbers in one shot? I'll have a heart attack!' In reality, the Free Roulette System #1 offers a 28.8% advantage over playing singular numbers in long sessions. That's mathematics, and there is no heart to worry about, axiomatic one.
You can also use SuperFormula to calculate all kinds of probabilities and advantage percentages. The option L — At least M successes in N trials is a very useful gambling instrument. If you play 19 numbers in one spin, the probability to win is 50%. If you play 19 numbers in 2 consecutive spins, the probability to win at least once is 75%.
Editor's note
• In an apparent change of heart, the Hamburg casino (Spielbank) offers online roulette results for free. The new link is (for the time being!):
www.spielbank-hamburg.de/spielsaal/permanenzen.php4
• • Real-life roulette spins are also available from the Wiesbaden, Germany, Casino (Spielbank)
www.spielbank-wiesbaden.de/DE/621/Permanenzen2.php: Wiesbaden Spielbank Permanenzen
Roulette: Software, Systems, Super Strategy
See a comprehensive directory of the pages and materials on the subject of roulette, software, systems, and the Super Strategy.- Theory, Mathematics of Roulette Systems, Strategies, Software.
- The Best-Ever Roulette Strategy, Systems based on mathematics of progressions and free-for-all.
- An inventory of free and outrageously pricedroulette systems.
- Software, Systems: Roulette Wheel Positioning, Sectors, Birthday Paradox.
- 'Roulette System that Won Millions!'
~ Ion Saliu's roulette theory, systems, and software were pirated by an Australian gambling group. - James Bond Roulette System for Dozens, Columns.
- Roulette Systems, Threats from Casino Chairman.
- Anti-Gambler Advice from John Patrick, Casino Moleextraordinaire and conspirator.
- Wizard of Odds Had High Praise For Ion Saliu's Gambling Theory.
- Casinos pay troubled individuals to intimidate intelligent gambling system authors.
- Download roulette, blackjack, casino gambling, systems, software.
| Home | Search | New Writings | Odds, Generator | Contents | Forums | Sitemap |

